Understanding LF Headroom in Arrays
Modern line array elements have very narrow vertical dispersion at high frequencies, which allows us to manipulate inter-element splay angles to manage overlap and therefore power addition. However, at LF, dispersion is very wide (approaching omnidirectional) and the elements overlap regardless of splay angle. Thus, all boxes in an array contribute to LF headroom over the coverage area, whereas at HF, headroom for a particular part of the listening area is affected only by the number of boxes aimed there.
As a thought experiment, let’s think about going from a single box to four boxes. We can control the HF addition, from total (100% overlap) to none (0% overlap, or “unity splay”). However, in all cases we should expect that the LF will see a 12 dB increase (20log(4/1)) or two doublings of quantity, so 6 dB + 6 dB, assuming the elements are in phase.
This is exactly what happens: Here is a measurement of a 4 box ground stack at 2° splays, vs a single box with the other three muted.
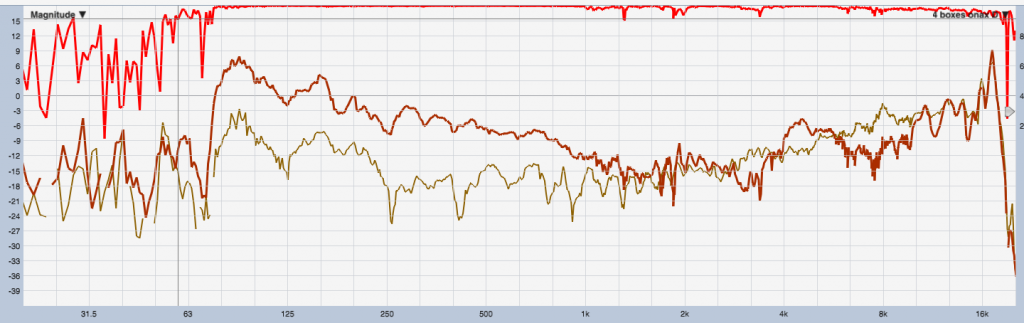
We see a full 12 dB increase at the low end of the response, and the summation gradually falls off as frequency increases and box coverage becomes more narrow (less overlap). Once we reach 2 kHz or, the HF waveguide has full control and the boxes are significantly less overlapped, so the HF response is dominated by the single box pointed at the microphone.
This is why level shading / per box EQ is extremely effective at HF, and a fool’s errand at 200 Hz.